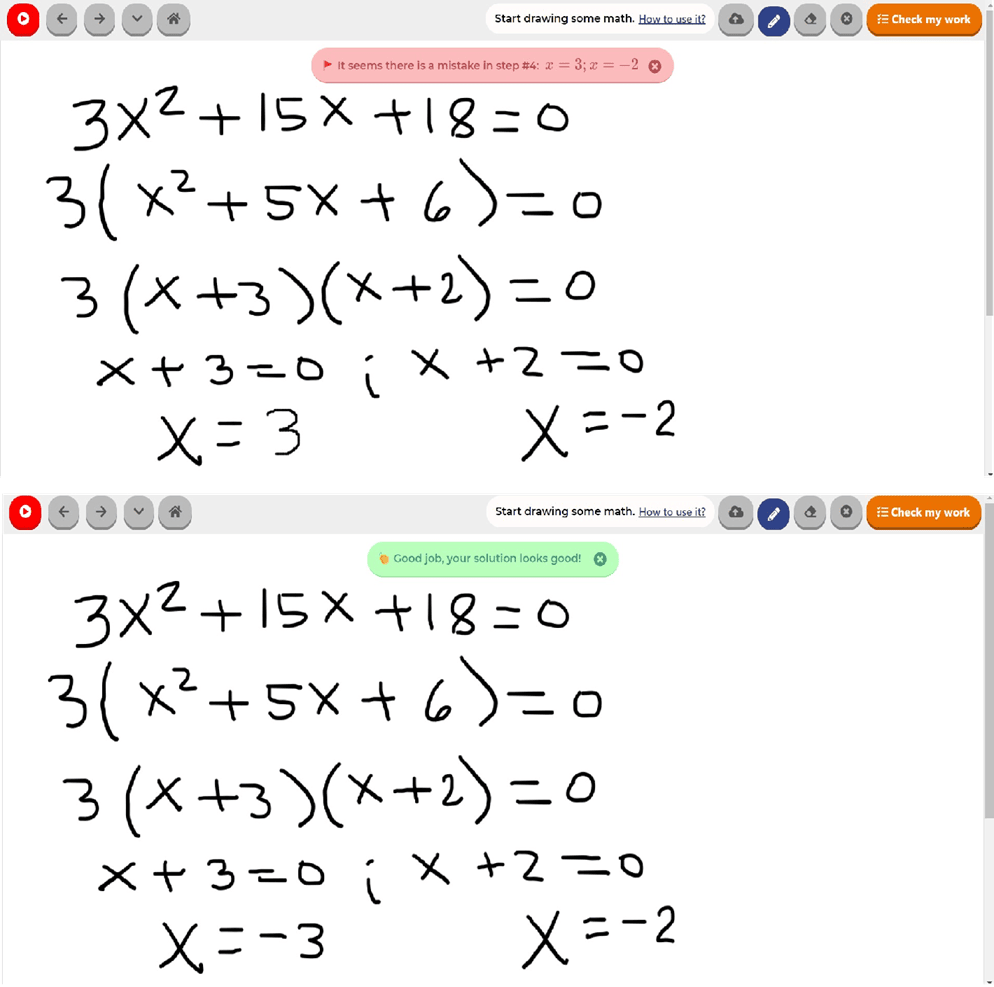
Risposta finale al problema
Soluzione passo-passo
Come posso risolvere questo problema?
- Scegliere un'opzione
- Sostituzione di Weierstrass
- Prodotto di binomi con termine comune
- Per saperne di più...
Applicare la formula: $\int\sin\left(\theta \right)^ndx$$=\frac{-\sin\left(\theta \right)^{\left(n-1\right)}\cos\left(\theta \right)}{n}+\frac{n-1}{n}\int\sin\left(\theta \right)^{\left(n-2\right)}dx$, dove $n=4$
Impara online a risolvere i problemi di passo dopo passo.
$\frac{-\sin\left(x\right)^{3}\cos\left(x\right)}{4}+\frac{3}{4}\int\sin\left(x\right)^{2}dx$
Impara online a risolvere i problemi di passo dopo passo. int(sin(x)^4)dx. Applicare la formula: \int\sin\left(\theta \right)^ndx=\frac{-\sin\left(\theta \right)^{\left(n-1\right)}\cos\left(\theta \right)}{n}+\frac{n-1}{n}\int\sin\left(\theta \right)^{\left(n-2\right)}dx, dove n=4. Moltiplicare il termine singolo \frac{3}{4} per ciascun termine del polinomio \left(\frac{1}{2}x-\frac{1}{4}\sin\left(2x\right)\right). L'integrale \frac{3}{4}\int\sin\left(x\right)^{2}dx risulta in: \frac{1}{2}\cdot \frac{3}{4}x-\frac{1}{4}\cdot \frac{3}{4}\sin\left(2x\right). Raccogliere i risultati di tutti gli integrali.