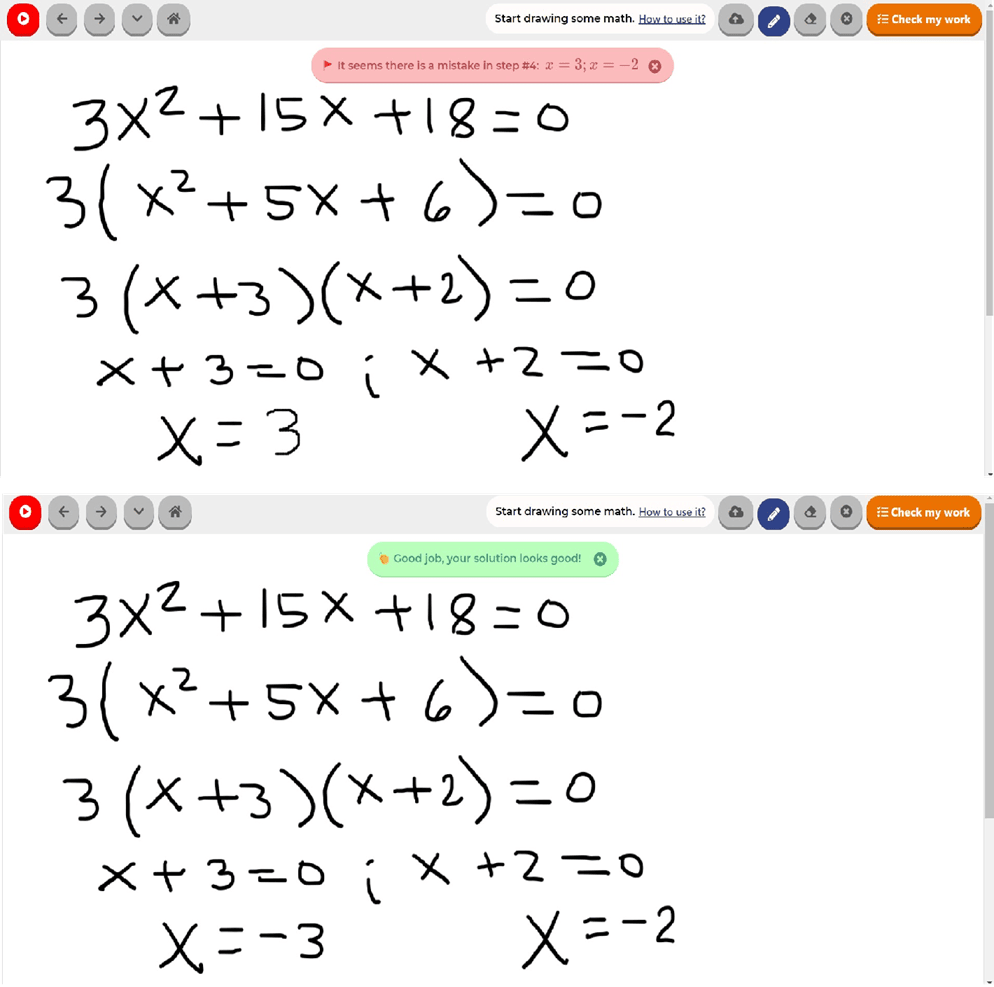
Risposta finale al problema
Soluzione passo-passo
Come posso risolvere questo problema?
- Scegliere un'opzione
- Scrivere nella forma più semplice
- Semplificare
- Fattore
- Trovare le radici
- Per saperne di più...
Applicare la formula: $\left(a+b\right)\left(a+c\right)$$=a^2-b^2$, dove $a=\sin\left(x\right)$, $b=\cos\left(x\right)$, $c=-\cos\left(x\right)$, $a+c=\sin\left(x\right)+\cos\left(x\right)$ e $a+b=\sin\left(x\right)-\cos\left(x\right)$
Impara online a risolvere i problemi di fattore per differenza dei quadrati passo dopo passo.
$f\left(x\right)=\sin\left(x\right)^2-\cos\left(x\right)^2+2\cos\left(2x\right)$
Impara online a risolvere i problemi di fattore per differenza dei quadrati passo dopo passo. f(x)=(sin(x)-cos(x))(sin(x)+cos(x))+2cos(2x). Applicare la formula: \left(a+b\right)\left(a+c\right)=a^2-b^2, dove a=\sin\left(x\right), b=\cos\left(x\right), c=-\cos\left(x\right), a+c=\sin\left(x\right)+\cos\left(x\right) e a+b=\sin\left(x\right)-\cos\left(x\right). Applying the trigonometric identity: \sin\left(\theta \right)^2-\cos\left(\theta \right)^2 = -\cos\left(2\theta \right). Combinazione di termini simili -\cos\left(2x\right) e 2\cos\left(2x\right).