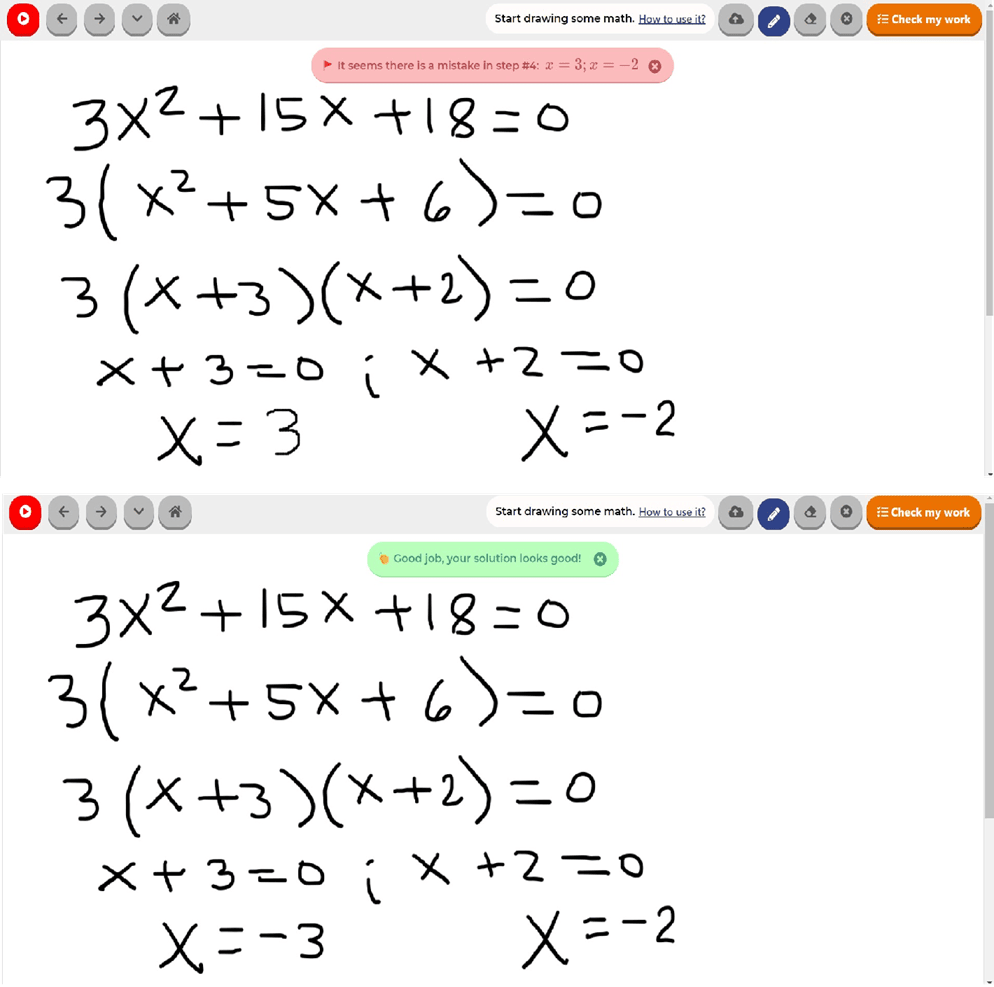
Risposta finale al problema
Soluzione passo-passo
Come posso risolvere questo problema?
- Scegliere un'opzione
- Prodotto di binomi con termine comune
- Metodo FOIL
- Sostituzione di Weierstrass
- Dimostrare dal LHS (lato sinistro)
- Per saperne di più...
Applicare la formula: $\frac{a}{b}$$=\frac{a}{b}\frac{radicalfactor\left(b\right)}{radicalfactor\left(b\right)}$, dove $a=10$ e $b=\sqrt{2}$
Impara online a risolvere i problemi di razionalizzazione passo dopo passo.
$\frac{10}{\sqrt{2}}\cdot \frac{\sqrt{2}}{\sqrt{2}}$
Impara online a risolvere i problemi di razionalizzazione passo dopo passo. Rationalize and simplify the expression 10/(2^(1/2)). Applicare la formula: \frac{a}{b}=\frac{a}{b}\frac{radicalfactor\left(b\right)}{radicalfactor\left(b\right)}, dove a=10 e b=\sqrt{2}. Applicare la formula: \frac{a}{b}\frac{c}{f}=\frac{ac}{bf}, dove a=10, b=\sqrt{2}, c=\sqrt{2}, a/b=\frac{10}{\sqrt{2}}, f=\sqrt{2}, c/f=\frac{\sqrt{2}}{\sqrt{2}} e a/bc/f=\frac{10}{\sqrt{2}}\cdot \frac{\sqrt{2}}{\sqrt{2}}. Applicare la formula: x\cdot x=x^2, dove x=\sqrt{2}. Applicare la formula: \left(x^a\right)^b=x, dove a=\frac{1}{2}, b=2, x^a^b=\left(\sqrt{2}\right)^2, x=2 e x^a=\sqrt{2}.