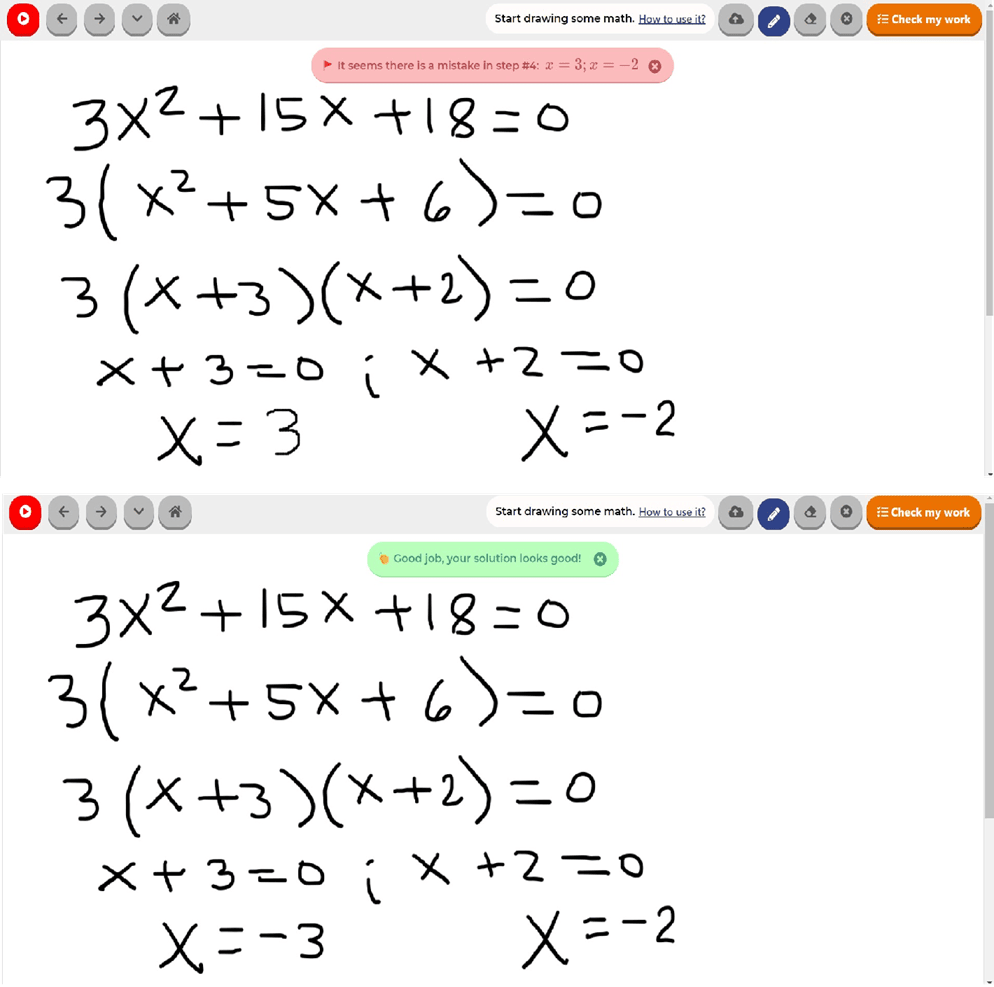
Risposta finale al problema
Soluzione passo-passo
Come posso risolvere questo problema?
- Scegliere un'opzione
- Semplificare
- Fattore
- Trovare le radici
- Trovare i punti di pareggio
- Per saperne di più...
Esprimere i numeri dell'equazione come logaritmi in base $2$
Impara online a risolvere i problemi di equazioni trigonometriche passo dopo passo.
$\log_{2}\left(32\right)=\log_{2}\left(2^{5}\right)$
Impara online a risolvere i problemi di equazioni trigonometriche passo dopo passo. Solve the equation log2(32)=5. Esprimere i numeri dell'equazione come logaritmi in base 2. Applicare la formula: \log_{a}\left(x\right)=\log_{a}\left(y\right)\to x=y, dove a=2, x=32 e y=2^{5}. Applicare la formula: a^b=a^b, dove a=2, b=5 e a^b=2^{5}. Applicare la formula: a=b=vero, dove a=32, b=32 e a=b=32=32.