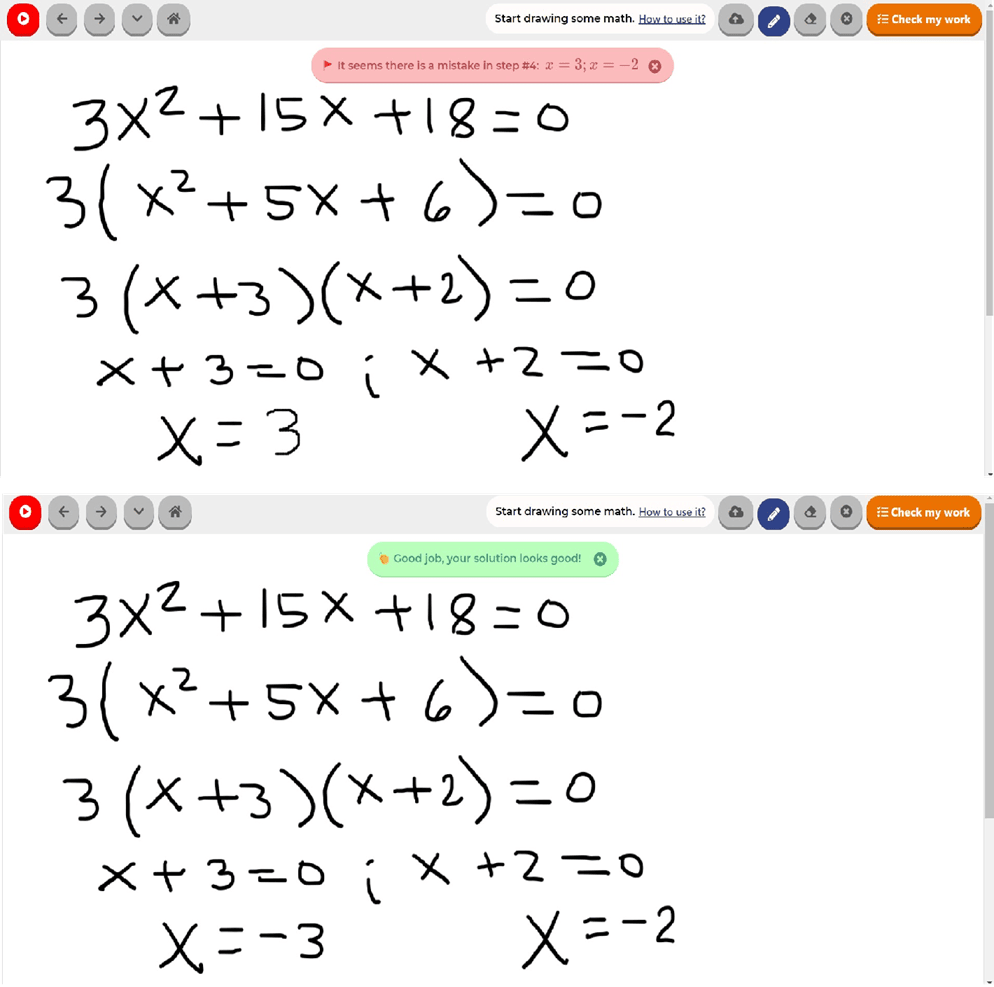
Risposta finale al problema
Soluzione passo-passo
Come posso risolvere questo problema?
- Scegliere un'opzione
- Risolvere per x
- Semplificare
- Fattore
- Trovare le radici
- Per saperne di più...
Applicare la formula: $x^a$$=\left(x^a\right)^{coef\left(a\right)}$, dove $a=2x$, $x=e$ e $x^a=e^{2x}$
Impara online a risolvere i problemi di equazioni esponenziali passo dopo passo.
$\left(e^x\right)^2-e^x-6=0$
Impara online a risolvere i problemi di equazioni esponenziali passo dopo passo. Solve the exponential equation e^(2x)-e^x+-6=0. Applicare la formula: x^a=\left(x^a\right)^{coef\left(a\right)}, dove a=2x, x=e e x^a=e^{2x}. Possiamo provare a fattorizzare l'espressione \left(e^x\right)^2-e^x-6 applicando la seguente sostituzione. Sostituendo il polinomio, l'espressione risulta essere. Fattorizzare il trinomio u^2-u-6 trovando due numeri che si moltiplicano per formare -6 e la forma addizionale -1.